NCERT Solutions for Class 11 Maths Chapter 1 Sets Ex 1.5
Get Free NCERT Solutions for Class 11 Maths Chapter 1 Sets Ex 1.5 PDF in Hindi and English Medium. Sets Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. Sets Exercise 1.5 Class 11 Maths NCERT Solutions were prepared by Experienced LearnCBSE.in Teachers. Detailed answers of all the questions in Chapter 1 Class 11 Sets Ex 1.5 provided in NCERT Textbook.
- Class 11 Sets Ex 1.1
- Class 11 Sets Ex 1.1 in Hindi Medium
- Class 11 Sets Ex 1.2
- Class 11 Sets Ex 1.2 Hindi Medium
- Class 11 Sets Ex 1.3
- Class 11 Sets Ex 1.3 Hindi Medium
- Class 11 Sets Ex 1.4
- Class 11 Sets Ex 1.4 Hindi Medium
- Class 11 Sets Ex 1.5
- Class 11 Sets Ex 1.5 Hindi Medium
- Class 11 Sets Ex 1.6
- Class 11 Sets Ex 1.6 Hindi Medium
- Class 11 Sets Miscellaneous Exercise
- Class 11 Sets Miscellaneous Exercise in Hindi Medium
- Sets Class 11 Notes Maths Chapter 1
- NCERT Exemplar Class 11 Maths Chapter 1 Sets
- RD Sharma Class 11 Solutions Chapter 1 Sets
- Chapter 1: Sets Class 11 NCERT Book
- अध्याय 1: समुच्चय Class 11 NCERT Book
Free download NCERT Solutions for Class 11 Maths Chapter 1 Sets Ex 1.5 PDF in Hindi Medium as well as in English Medium for CBSE, Uttarakhand, Bihar, MP Board, Gujarat Board, BIE, Intermediate and UP Board students, who are using NCERT Books based on updated CBSE Syllabus for the session 2019-20.
Class 11 Maths NCERT Solutions Chapter 1 Sets Ex 1.5
Q.1: Let U = {1, 2, 3, 4, 5, 6, 7, 8, 9}
X = {1, 2, 3, 4, 5}
Y = {2, 4, 6} and
Z = {2, 4, 5, 6}.
Find the following sets:
(i). X’
(ii). Y’
(iii). (X∪Y)′
(iv). (X∪Z)′
(v). (X′)‘
(vi). (Y–Z)′
Solution:
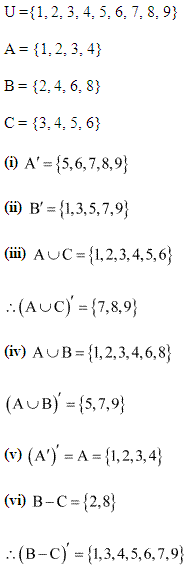
Q.2: If U = {a, b, c, d, e, f, g, h}, find the complements of the given sets:
(i). W = {a, b, c}
(ii). X = {d, e, f, g}
(iii). Y = {a, c, e, g}
(iv). Z = {f, g, h, a}
Solution:

Q.3: Take natural numbers as the universal set. Write the complements of the given sets:
(i). A = {y: y is an even natural number}
(ii). B = {y: y is an odd natural number}
(iii). C = {y: y is a positive multiple of 3}
(iv). D = {y: y is a prime number}
(v). E = {y: y is a natural number divisible by 3 and 5}
(vi). F = {y: y is a perfect square}
(vii). G = {y: y is perfect cube}
(viii). H = {y: y + 5 = 8}
(ix). I = {y: 2y + 5 = 9}
(x). J = {y: y ≥ 7}
(xi). K = {y: y ∈ N and 2y + 1 > 10}
Solution:
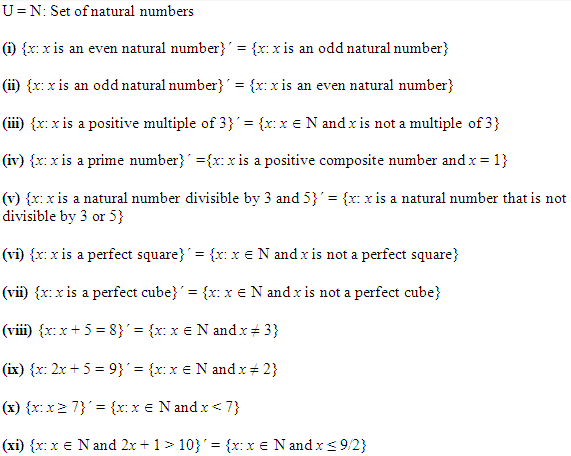
Q.4: If U = {1, 2, 3, 4, 5,6,7,8, 9}
A = {2, 4, 6, 8} and, B = {2, 3, 5, 7}.
Verify that:
(i). (A∪B)′ = A′∩B′
(ii). (A∩B)′ = A′∪B′
Solution:
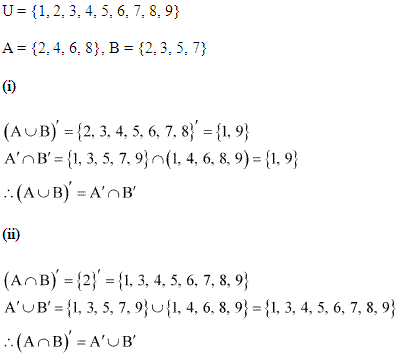
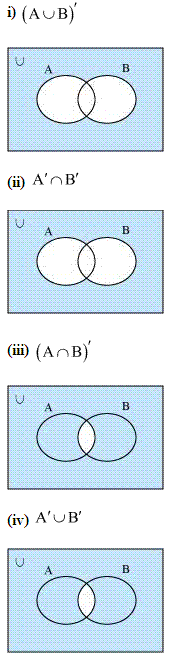
Q.5: Draw the Venn diagrams for the following:
(i). (A∪B)′
(ii). A′∩B′
(iii). (A∩B)′
(iv). A′∪B′
Solution:
Q.6: Let U be the universal set that is the set of all triangles in a plane. If X is the set of all triangles with at least one angle different from 60∘, what is X’ ?
Solution:
![]()
Q.7: Complete the given statements using proper symbols:
(i). A∪A′
(ii). Ø ∩A
(iii). A∩A′
(iv). U′∩A
Solution:
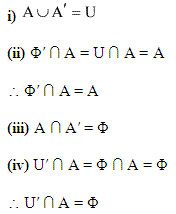
NCERT Solutions for Class 11 Maths Chapter 1 Sets Ex 1.5 in Hindi

प्रश्न 3.
प्राकृत संख्याओं के समुच्चय को सार्वत्रिक समुच्चय मानते हुए, निम्नलिखित समुच्चयों के पूरक लिखिए:
(i) {x : x एक प्राकृत सम संख्या है।}
(ii) {x : x एक प्राकृत विषम संख्या है।}
(iii) {x : x संख्या 3 को एक धन गुणज है।}
(iv) {x : x एक अभाज्य संख्या है।}
(v) {x : x, 3 और 5 से विभाजित होने वाली एक संख्या है।}
(vi) {x : x एक पूर्ण वर्ग संख्या है।}
(vii) {x : x एक पूर्ण घन संख्या है।}
(viii){x : x + 5 = 8}
(ix) {x : 2x + 5 = 9}
(x) {x : x ≥ 7}
(xi) {x : x ∈ N और 2x + 1 > 10}
हल:
(i) {x : x एक विषम प्राकृत संख्या है।}
(ii) {x : एक सम संख्या है।}
(iii) {x : x ∈ N और x संख्या 3 का धन गुणज नहीं है।}
(iv) {x : x = 1 और x एक धन भाज्य संख्या है।}
(v) {x : x ∈ N और x, संख्या 3 व 5 किसी से भी विभाजित नहीं होती।}
(vi) {x : x ∈ N तथा x एक पूण वर्ग संख्या नहीं है।}
(vii) {x : x ∈ N तथा x एक पूर्ण वर्ग घन संख्या नहीं है।}
(viii) {x : x ∈ N तथा x ≠ 3}
(ix) {x : x ∈ N तथा x ≠ 2}
(x) {x : x ∈ N तथा x < 7}
(xi) {x : x ∈ N तथा x < \(\frac { 9 }{ 2 }\)}
प्रश्न 4.
यदि U = {1, 2, 3, 4, 5, 6, 7, 8, 9}, A = {2, 4, 6, 8} और B = {2, 3, 5, 7}, तो सत्यापित कीजिए कि:
(i) (A ∪ B)’ = A’ ∩ B’
(ii) (A ∩ B)’ = A’ ∪ B’
हल:
(i) A ∪ B = {2, 4, 6, 8} ∪{2, 3, 5, 7} = {2, 3, 4, 5, 6, 7, 8}
बायाँ पक्ष = (A ∪B)’ = U – (A ∪ B) = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2, 3, 4, 5, 6, 7, 8} = {1, 9}
A’ = U – A = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2, 4, 6, 8} = {1, 3, 5, 7, 9}
B’ = U – B = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2, 3, 5, 7} = {1, 4, 6, 8, 9}
दायाँ पक्ष = A’ ∩ B’ = {1, 3, 5, 7, 9} ∩ {1, 4, 6, 8, 9} = {1, 9}
अतः (A ∪ B)’ = A’ ∩ B’.
(ii) बायाँ पक्ष = (A ∩B)’
(A ∩B) = {2, 4, 6, 8} ∩ {2, 3, 5, 7} = {2}
(A ∩ B)’ = {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2} = {1, 3, 4, 5, 6, 7, 8, 9}
दायाँ पक्ष : A’ ∪ B’ = {1, 3, 5, 7, 9} ∪ {1, 4, 6, 8, 9} = {1, 3, 4, 5, 6, 7, 8, 9}
अत: (A ∩ B)’ = A’ ∪ B’.
प्रश्न 5.
निम्नलिखित में से प्रत्येक के लिए उपयुक्त वेन आरेख खींचिए।
(i) (A ∪ B)’
(ii) A’ ∩ B’
(iii) (A ∩B)’
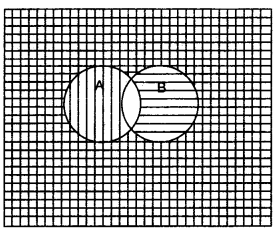
(iv) (A’ ∪ B’)
हल:
छायांकित क्षेत्र को निम्नलिखित समुच्चयों द्वारा दर्शाते हैं:
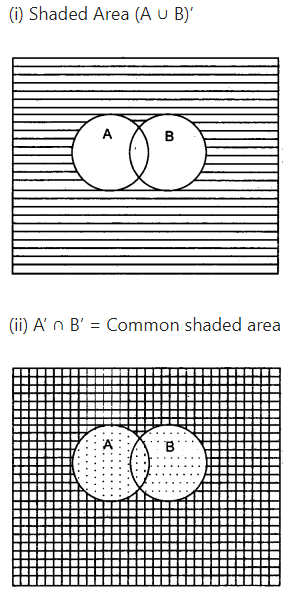
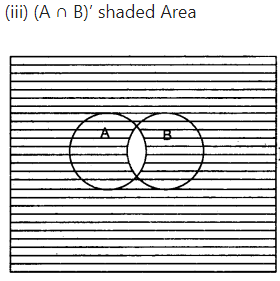
(iv) (A’ ∪ B’)
प्रश्न 6.
मान लीजिए कि किसी समतल में स्थित सभी त्रिभुजों का समुच्चय सार्वत्रिक समुच्चय U है। यदि A उन सभी त्रिभुजों का समुच्चय हैं जिनमें कम से कम एक कोण 60° से भिन्न है, तो A’ क्या है?
हल:
U = {x : समतल में एक त्रिभुज है।}
A = {x : x एक त्रिभुज जिसका कम से कम एक कोण 60° का न हो।}
A’ = {सभी समबाहु त्रिभुजों का समुच्चय है।}
प्रश्न 7.
निम्नलिखित कथनों को सत्य बनाने के लिए रिक्त स्थान भरिए:
(i) A ∪ A’ = ………..
(ii) Φ’ ∩ A = ………..
(iii) A ∩A’ = ………….
(iv) U’ ∩ A = …………
हल:
(i) A ∪ A’ = U
(ii) Φ’ ∩ A = U ∩ A = A
(iii) A ∩A’ = Φ
(iv) U’ ∩ A = Φ ∩ A = Φ