Students can use Maths Mela Class 3 Solutions Chapter 12 Give and Take Question Answer to explore alternative problem-solving methods.
Class 3 Maths Chapter 12 Give and Take Question Answer Solutions
Give and Take Class 3 Maths Question Answer
Class 3 Maths Chapter 12 Solutions

Answer:

Kishan runs a big plant nursery where he puts different varieties of plants. Villagers often come and take saplings from him to grow in their houses.

1. Kishan had 364 saplings of different herbs and flowers. Then he went to his friend’s village and brought 52 saplings from there. How many saplings does he have now?
Let us draw a box diagram for the problem.

364 saplings + 52 saplings = _____ saplings
364 + 52 = ______
You can also use a number line to solve it.

Answer:
![]()
2. Kishan has got an order to deliver 230 saplings to a school. He has packed 75 saplings in an open box. How many more saplings does he need to pack?
We write the given problem as a box diagram:

Subtracting 5 ones from 10 ones, we are left with 5 ones.
Now we have to take away 70. Remember opening a hundreds block gives us 10 blocks of 10s.


Kishan has …………. saplings now.
Let us Do
Draw box diagrams, as shown above to solve the following problems. Then use HTO blocks or a number line to solve the problems.
Question 1.
Kishan has 456 saplings in August. He distributed 63 saplings. How many saplings are left with him?
Answer:
Box Diagram


Question 2.
Kishan has a collection of 309 saplings. He gets 80 more saplings of flowering plants. How many saplings does he have now?
Answer:
Box diagram

![]()
Question 3.
Kishan has 270 saplings of herbs and his friend has 36 saplings of herbs. How many more saplings does Kishan have than his friend?
Answer:
Box diagram

• Write word problems using the numbers given in the box diagrams below and solve them. You can take help from the pictures for appropriate contexts.


Answer:

• There are 234 boys and 156 girls in a school. How many total students are in the school?
Answer:

• In a library there are 356 books in which 138 books are for children. How many are not for children?
Answer:

• In a truck 305 sacks can be load. If 210 sacks have been loaded than how many more sacks can be loaded?
Answer:

• Use the grid below to solve the following questions. Colour your answers in the grid.

Answer:

Do as directed.

Answer:

Many years ago, in the Village ‘Jadupur’, people exchanged things based on their need. Shaamu Kaka gave 5 sacks of rice to Dariya Didi. She in return gave 10 sacks of vegetables. Dariya Didi got 2 sarees from Bablu Dada by giving 5 sacks of onions.

Like this, people in the village exchanged their things. Shamu Kaka got vegetables for the rice he gave. Dariya Didi gave lots of onions to Bablu Dada for the two sarees. Discuss in class why people in this village had to give different quantities while exchanging things.

These days we use money in exchange for things we need. Notes and coins come in different values which are used to buy different things.
For example, one 10-rupee note can buy one Hawa Mithai or ten toffees.

One Hawa Mithai costs more than a toffee.
Salma buys two bottles of milk for ₹ 100. Kiran buys a basket of pomegranates for ₹ 100.
Circle the one that costs more: a milk bottle or a pomegranate?

• Think of two things that we can buy using the same note.

Answer:

• Match the notes and coins in the two columns that have the same values.

Answer:

Use the following notes and coins to buy the things given below. Find at least two ways of giving the money. You may use the notes and coins more than once.

Answer:

![]()

Answer:
•

• 5
• We can buy a dress or school bag or a pair of shoes or some big packets of chocolates with ₹ 500.

In the morning, Peter uncle has ₹ 465 in his money box. By afternoon, he has ₹ 756. How much has he earned since morning?

• Today, Peter uncle sold rice for ₹ 640 and sugar for ₹ 215. How much money has he earned from this sale?


Answer:

•

Answer:

• Combining both money, we got
Answer:

• Solve by using number line.
Answer:

Let us Do
Solve the following problems using box diagrams. Estimate the answers. Then use notes of ₹ 100s, ₹ 10s and ₹1 is or a number line to solve the problems.
Question 1.
One day Peter uncle earned ₹ 650. The next day he earned ₹ 250 more. How much money had he earned by the second day?
Answer:

Question 2.
Reena bought groceries for ₹ 209. She gave a ₹ 500 note to Peter uncle. How much money should Peter uncle return to Reena?
Answer:

![]()
Question 3.
Shireen has ₹ 150 in her piggy bank. She puts ₹ 1oo every week in her piggy bank. How much money does she have at the end of four weeks?
Answer:

Question 4.
Peter uncle saved ₹ 250 in the first month, ₹ 125 in the second month and ₹ 350 in the third month. How much has he saved in these three months?

Answer:

• Estimate the answers to the nearest hundred. Share your thinking in the class.

Answer:

• Compare the given problem statements in each row, without calculating. Circle the one that is more. Share your thinking in class.

Answer:

• Find the pairs that are equal. Share your thinking in class.

Answer:

• Fill in the boxes with appropriate numbers.

Answer:

Make cards with numbers 0-5. Make two 3-digit numbers using these cards. Add the two numbers and subtract the two numbers. Rearrange the cards and try to get a bigger sum. Rearrange the cards and try to get a smaller difference.
Check with your friends who has got the biggest sum and smallest difference.

Let us Do
(a) 265 + 9
Answer:
265 + 9 = 274
(b) 405 + 56
Answer:
405 + 56 = 461
(c) 825 + 175
Answer:
825 + 175 = 1000
(d) 600 – 82
Answer:
600 – 82 = 518
(e) 568 – 5
Answer:
568 – 5 = 563
(f) 653 – 356
Answer:
653 – 356 = 297
NCERT Solutions for Class 3 Mathematics Chapter 12 Can We Share? (Old Syllabus)
Share the Grains
1.Mummy bird brings 12 grains.
How to distribute equally?
Mummy bird starts by giving 1 grain to each baby. Then mummy bird gives one more grain to each baby. Each baby has got 2 grains now. How many grains are
Ans. There are 4 baby birds and each baby bird gets 2 grains.
So, number of grains with baby birds = 2×4 = 8
So, number of grains left = 12 – 8 = 4
She puts one more grain in each baby’s mouth. All the grains are finished.
12 grains have been divided among 4 baby birds. Each baby has got 3 grains. 12÷4 = 3
Try These Now
Gopu has 3 plates of jalebis.
Each plate has a different number of jalebis.
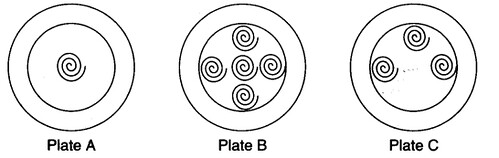
Now draw the jalebis on the plates below, so that each plate has the same number of jalebis.
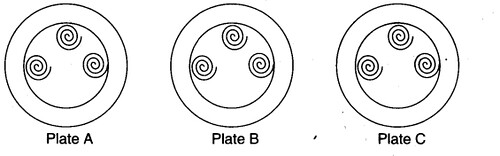
1.How many jalebis are there altogether?
Ans. 1 + 5 + 3 = 9 jalebis.
2.How many jalebis are there in each plate?
Ans. 3 jalebis.
3.Discuss in the class how you found the answer.
Ans. Total number of jalebis = 1 + 5 + 3 = 9
Total number of jalebis is divided into 3 equal parts:
9÷3 = 3
So, each plate gets 3 jalebis.
Sharing Them Equally?
Here are six bananas.
Here are three monkeys.
If they share the bananas equally, each monkey will get 2 bananas.
6 bananas divided into 3 equal parts = 2 bananas each 6 + 3 = 2
If there are six bananas and two monkeys,
Each monkey will get three bananas.
Six bananas + 2 = 3 bananas each.
6 ÷2 = 3
1.If there are 60 bananas and two monkeys, how many will each monkey get?
Ans. 60 ÷ 2 = 30 bananas
So, each monkey will get 30 bananas.
2.What if there are 600 bananas and two monkeys?
Ans. 600 ÷ 2 = 300 bananas
So, each monkey will get 300 bananas.

Five friends found 10 five-rupee coins on the ground. They shared them equally. Each friend got ten rupees.
50 ÷ 5 = 10
If there are 16 ten-rupee notes and four friends to share, then 16 ÷ 4 = 4 ten-rupee notes and 4 x 10 = 40. So, each friend gets 4 x 10 = 40
rupees.
1.Five friends found ? 100. If they share it equally, how much will each get?
Ans.100÷ 5 = 20.
2. Hari Prasad has 30 metres of rope.
He distributes it equally among his three children. Each child gets———–metres of rope.
Ans. 30÷3 = 10 metres.
3.If there is 36 metres of rope, how much of rope will each child get?
Ans. 36 ÷ 3 = 12 metres.
4.If there is 60 metres of rope, how much will each child get?
Ans. 60 ÷ 3 = 20 metres.
How Many Shelves?
1.I have 20 books. I can keep 5 books in one shelf, so how many shelves do I need in my almirah?
Ans. 20÷5 = 4 shelves.
Practice Time
1. Minku puts her 15 laddoos equally into 5 boxes.
(i)How many laddoos will there be in each box?
Ans. 15 ÷ 5 = 3 laddoos in each box.
(ii)If she uses only 3 boxes, how many laddoos will there be in each box?
Ans. 15 ÷ 3 = 5 laddoos in each box.
2.Share 25 bananas among 5 monkeys. How many bananas for each monkey?
Ans. 25 ÷5 = 5 bananas for each monkey.
3.Share 12 balloons among 3 boys. How many balloons for each boy?
Ans. 12÷ 3 = 4 balloons for each boy.
4.There are 21 candles. Put them equally in 3 boxes. How many candles are there in each box?
Ans. 21 ÷ 3 = 7 candles in each box.
5.There are 18 socks. How many girls can wear these socks?
Ans. One girl wears 2 socks.
So, 18÷ 2 = 9 girls can wear these socks.
Raj has 36 minutes to make rotis. One roti takes 3 minutes.
6.How many rotis can he make in this time?
Ans. 36 ÷ 3 = 12
So, Raj can make 12 rotis in this time.
7.There are 24 foot marks of goats. So, how many goats are there?
Ans. 1 goat will make 4 foot marks , So, 24 -r 4 = 6 goats are there.
8.Some girls are playing a game with both their hands. The girls,“who are playing, ! have 60 fingers; altogether. How many girls are playing this game?
Ans. Each girl has 10 fingers.
So, 60 ÷10 = 6 girls are playing this game.
9.Lakshmi has 27 kg potatoes to sell. Three men came and bought equal amounts————— of potatoes. Each man bought kg of potatoes.
Ans. 27 ÷ 3 = 9 kg of potatoes.
Jumpy Animals
1.In how many jumps will the frog reach 30?
Ans. 30÷2 = 15.
2.In how many jumps will the squirrel reach 27?
Ans. 27÷ 3 = 9.
3.Which number will the kangaroo reach in two jumps?
Ans. 30 x 2 = 60
4.Who all will meet at number 15?
Ans. Squirrel, rabbit, horse.
Explanation: One jump of squirrel is 3 steps and 15 is divisible by 3 One jump of the rabbit is 5 steps and 15 is divisible by 5 One jump of horse is 15 steps.
So, all these three animals can meet at number 15.
5.Will the rabbit ever be at the number 18?
Ans. No.
Explanation: One jump of rabbit is 5 steps and 18 is not divisible by 5. So, the rabbit can never reach number 18.
6.How many jumps of the rabbit equal one jump of the horse?
Ans. One jump of horse = 15 steps One jump of rabbit = 5 steps
So, 15÷ 5 = 3 jumps of rabbit equal one jump of the horse.
7.How’ many jumps of the horse equal two jumps of the kangaroo?
Ans. One jump of kangaroo = 30 steps
So, two jumps of kangaroo = 30 x 2 = 60 steps One jump of horse = 15 steps
So, 60 ÷15 = 4 jumps of horse equal two jumps of kangaroo.
8.Which is the smallest number where the frog and the squirrel will meet?
Ans. One jump of frog = 2 steps
One jump of squirrel = 3 steps And, 2×3 = 6
So; the smallest number where the frog and the squirrel will meet is 6.