Real Numbers Class 10 Extra Questions Maths Chapter 1 with Solutions

Extra Questions for Class 10 Maths Real Numbers with Answers

Extra Questions for Class 10 Maths Chapter 1 Real Numbers. According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

Real Numbers Class 10 Extra Questions Very Short Answer Type

Question 1.
After how many places of decimal, the decimal expansion of \(\frac{43}{2^4 \times 5^3}\) will terminate?
Answer:
Given \(\frac{43}{2^4 \times 5^3}\) is in the lowest form and power of 2 = 4, Power of 5 = 3, Max. {4, 3} = 4
∴ \(\frac{43}{2^4 \times 5^3}\) will terminate after 4 places of decimal.

Question 2.
What is the exponent of 3 in the prime factorisation of 864.
Answer:

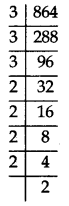
Making prime factors of 864. ,
⇒ 864 = 3 × 3 × 3 × 2 × 2 × 2 × 2 × 2
= 3³ × 2⁵
∴ Exponent of 3 in prime factorisation of 864 = 3.

Question 3.
Express 10010 and 140 as prime factors.
Answer:
Prime factors of 10010 = 2 × 5 × 7 × 11 × 13
Prime factors of 140 = 2 × 2 × 5 × 7

Question 4.
What is the H.C.F. of smallest prime number and the smallest composite number ? [CBSE 2018]
Answer:
Smallest prime number = 2 = 2¹
Smallest composite number = 4 = 2²
H.C.F. (2, 4) = 2¹ = 2

Question 5.
Write a rational number between √5 and √6.
Answer:
∵ √5 = 2.236… and √6 = 2.449…
∴ A rational no between 2.24 and 2.44 (approximately) is 2.3 or 2.31 or 2.32 etc.
Note: Take the lower limit slightly greater than √5 and upper limit slightly lesser than √6 .
⇒ One number between √5 and √6 = 2.3

Question 6.
If p,q are two prime numbers then what is the HCF and LCM of p and q?
Answer:
HCF (p, q) = 1
and LCM(p, q) = pq

Question 7.
A rational number in its decimal expansion is 623.6051. What can you say about the prime factors of q, when this number is expressed in the form \(\frac{p}{q}\)? Give reasons.
Answer:
Since 623.6051 is a terminating decimal number, so q must be of the form 2^m 5ⁿ where m, n are natural numbers.

Question 8.
‘Product of two irrational numbers is always an irrational number’. Negate the statement by giving counter example.
Answer:
Take 2 – √5, 2 + √5 both are irrational. Their product
= (2 – √5) (2 + √5)
= 2² – (√5)² = 4 – 5 = – 1
Which is a rational number.

Question 9.
Can two numbers have 24 as their HCF and 7290 as their LCM? Give reasons.
Answer:
No, because HCF always divide LCM but here 24 does not divide 7290.

Real Numbers Class 10 Extra Questions Short Answer Type-1

Question 1.
Find the HCF of 1260 and 7344 using Euclid’s algorithm.
Or
Show that every positive odd integer is of the form (4q + 1) or (4q + 3), where q is some integer. [CBSE 2019]
Answer:
Taking a = 7344 and b = 12260
Applying Euclid’s Division Algorithm

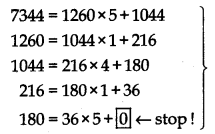
HCF of 1260 and 7344 is 36.
Or
Apply Euclid’s Division Lemma to a and b = 4.
a = 4q + r, 0 ≤ r < 4
⇒ a = 4q, a = 4q + 1, a = 4q + 2 and a = 4q + 3.
Now, a = 4q and a = 4q + 2 are even numbers as both are divisible by 2.
But, 4q + 1 and 4q + 3 are not divisible by 2.
Therefore, when a is odd, it is of the form
a = 4q + 1 or a = 4q + 3 for some integer q.

Question 2.
Show that \(\frac{3+\sqrt{7}}{5}\) that √7 is irrational.
Or
Prove that n² + n is divisible by 2 for any positive integer n. [CBSE 2019 (C)]
Answer:

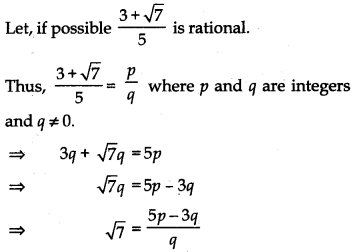
Since, difference of integers is also an integer.
∴ 5p – 3q is an integer.
⇒ \(\frac{5 p-3 q}{q}\) is rational number.
But LHS is √7, which is irrational.
Thus, irrational number = Rational number which is a contradiction.
Thus, our supposition is wrong.
Hence, \(\frac{3+\sqrt{7}}{5}\) is an irrational number.
Or
n² + n = n(n + 1)
There arise two cases.
Case I: When n is even.
Then (n +1) is odd.
Since, even × odd = even.
∴ Product n(n + 1) is even i.e. divisible by 2.

Case II: When n is odd.
Then (n + 1) is even.
Since, odd × even = even.
∴ n(n + 1) is even i.e. divisible by 2.
So, for any positive integer, (n² + n) is always divisible by 2.

Question 3.
A positive integer n when divided by 9, gives 7 as remainder. Find the remainder when (3n – 1) is divided by 9.
Answer:
Here n can be written as 9k + 7, where k ∈ N
Now 3n – 1 = 3(9k + 7) – 1 = 27k + 20
Applying Euclid’s division lemma on (27k + 20) and 9, we have
27k + 20 = 9 × (3k + 2) + 2;
where k ∈ N
Thus, 2 is the remainder.

Question 4.
Show that any positive even integer can be written in the form 6q, 6q + 2 or 6q + 4, where q is an integer? [CBSE Delhi 2016]
Answer:
Let take a¹ as any positive even integer and
b = 6
Then using Euclid algorithm we get
a = 6q + r
Here r is remainder and value of q is more than or equal to 0 and r = 0, 1, 2, 3, 4, 5 because 0 < r < b and the value of b is 6.
So total possible forms will be 6q + 0, 6q + 2, 6q + 4. 6q + 0
6 is divisible by 2 so, it is an even number. 6q + 2
6 is divisible by 2 and 4 is also divisible by 2.
So, it is an even number.
Hence, any positive even integer can be written in the form 6q, 6q + 2 or 6q + 4 and so on.

Question 5.
In a school, the duration of a period in junior section is 40 minutes and in senior section is 1 hour: If the first bell for each section ring at 9:00 a.m., when will the two bells ring together again? [CBSE 2012 (Sept.)]
Answer:
1 hour = 60 minutes
40 = 2 × 2 × 2 × 5 = 2³ × 5
60 = 2 × 2 × 3 × 5 = 2² × 3 × 5
∴ LCM (40, 60) = 2³ × 3 × 5 = 120
120 minutes = 2 hours
Hence, the two bells will ring together again at 9:00 + 2:00 = 11:00 a.m.

Question 6.
Explain why (17 × 11 × 2 + 17 × 11 × 5) is a composite number? [CBSE 2012 (Sept.)]
Answer:
17 × 11 × 2 + 17 × 11 × 5
= 17 × 11 × (2 + 5)
= 17 × 11 × 7
Since, 17 × 11 × 2 + 17 × 11 × 5 can be expressed as a product of primes, therefore, it is a composite number.

Real Numbers Class 10 Extra Questions Short Answer Type-2

Question 11.
Use Euclid’s division algorithm to find HCF of 4052 and 12576. [CBSE 2019 (C)]
Answer:
∵ 12576 > 4052, apply the Euclid’s division lemma to 12576 and 4052, to get
12576 = 4052 × 3 + 420
Since 420 ≠ 0, so apply E.D.L. to 4052 and 420 to get
4052 = 420 × 9 + 272
Since, 272 ≠ 0, so apply E.D.L. to 420 and 272
420 = 272 × 1 + 148
Since, 148 ≠ 0, so apply E.D.L. to 272 and 148 to get
272 = 148 × 1 + 124
Since, 124 ≠ 0, so apply E.D.L. to 148 and 124
148 = 124 × 1 + 24
Since, 24 ≠ 0, so apply E.D.L. to 124 and 24
124 = 24 × 5 + 4
Since, 4 ≠ 0, so apply E.D.L. to 24 and 4
24 = 4 × 6 + 0 ← Stop!
Since, remainder has become zero.
∴ HCF (12576, 4052) = 4.

Question 2.
The LCM of two numbers is 14 times their HCF. The sum of LCM and HCF is 600. If one number is 280, then find the other number.
[C.B.S.E Sept 2012]
Answer:
According to the statement of the question, we have:
LCM of two numbers = 14 × HCF of two numbers
Also, LCM + HCF = 600
⇒ 14 × HCF + HCF = 600
⇒ 15 HCF = 600
⇒ HCF = 40
∴ LCM = 14 × 40 = 560
Now, one number is 280
∴ 280 × Other number = 40 × 560
⇒ Other number = \(\frac{40 \times 560}{280}\) = 80

Question 3.
Show that any positive odd integer is of the form 6q + 1, 6q + 3 or 6q + 5, where q is some integer.
Answer:
Apply Euclid’s Division lemma to a and 6, we have
a = 6q + r, where 0 ≤ r ≤ 6
Thus, r can take values 0, 1, 2, 3, 4, 5.
Consider the equation, a = 3q + r
Case 1: When r = 0
Thus, a = 6q
Rewriting the above equation, we have
a = 2(3q)
Which is an even number.

Case 2: When r = 1
Thus, a = 6q + 1
Rewriting the above equation, we have
a = 2 × 3q + 1 – 2m + 1, where m = 3q
Which is an odd number.

Case 3: When r = 2
Thus, a = 6q + 2
Rewriting the above equation, we have
a = 2 × (3q + 1)
= 2m, where m = 3q + 1
Which is an even number.

Case 4: When r = 3
Thus, a = 6q + 3
Rewriting the above equation, we have
a = 2 × (3q + 1) + 1
= 2m + 1, where m = 3q + 1
Which is an odd number.

Case 5: When r = 4
Thus, a = 6q + 4
Rewriting the above equation, we have
a = 2 × (3q + 2)
= 2m + 1, where m = 3q + 2
Which is an even number.

Case 6: When r = 5
Thus, a = 6q + 5
Rewriting the above equation, we have
a = 2 × (3q + 2) + 1
= 2m + 1, where m = 3q + 2
Which is an odd number.
Therefore, any positive odd integer is of the form 6q + 1, 6q + 3 or 6q + 5, where q is some integer.

Question 4.
Using Euclid’s division algorithm, find HCF of 56, 96 and 404.
Answer:
Applying Euclid’s division algorithm to 56 and 96
96 = 56 × 1 + 40
56 = 40 × 1 + 16
40 = 16 × 2 + 8
16 = 8 × 2 + 0
∴ HCF (56, 96) = 8
Next, apply Euclid’s division algorithm to 8 and 404
404 = 8 × 50 + 4
8 = 4 × 2 + 0
Thus, HCF (56, 96, 404) = 4

Question 5.
Find HCF and LCM of 404 and 96 and verify that HCF × LCM = Product of the two given numbers. [CBSE 2018]
Answer:
404 = 2 × 2 × 101 = 2² × 101
96 = 2 × 2 × 2 × 2 × 2 × 3 = 25 × 3
∴ HCF of 404 and 96 = 2² = 4
[Product of smallest power of each common factor]
LCM of 404 and 96 = 101 × 2⁵ × 3 = 9696
[Product of greatest power of each prime factor]
HCF × LCM = 4 × 9696 = 38784
Also 404 × 96 = 38784
Hence HCF × LCM = Product of 404 and 96.

Question 6.
Express 5.4\(\overline{178}\) in the \(\frac{p}{q}\) for,.
Answer:
Let x = 5.4\(\overline{178}\)
or x = 5.4178178178 ………
∴ 10x = 54.178178178 ………. (1)
Also 1000 (10x) = 54178.178178178 …….
⇒ 10000x = 54178.178178178 ……………. (2)
Subtracting (1) from (2), we have:
10000x – 10x = [54178.178178178…] – [54.178178…]
⇒ 9990x = 54124

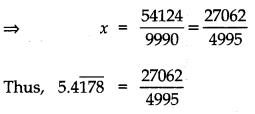
Question 7.
Find the LCM and HCF of 1296 and 5040 by prime factorisation method:
Answer:

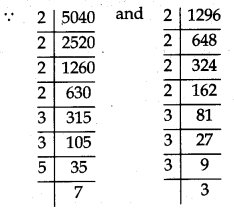
∴ 5040 = 2 × 2 × 2 × 2 × 3 × 3 × 5 × 7
= 2⁴ × 3² × 5 × 7
1296 = 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3
= 2⁴ × 3⁴
∴ LCM = Product of each prime factor with highest powers
= 2⁴ × 3⁴ × 5 × 7
= 16 × 81 × 5 × 7 = 45360
HCF = Product of common prime factors with lowest powers
= 2⁴ × 3²
= 16 × 9 = 144

Question 8.
The decimal expansion of the rational number \(\frac{43}{2^4 \times 5^3}\), will terminate after how many places of decimal?
Answer:

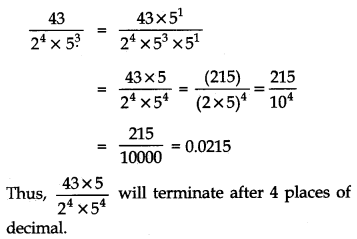
Question 9.
Show that any positive odd integer is of the form 4q + 1 or 4q + 3 where q is a positive integer.
Answer:
Let a be any positive integer and b = 4. Applying Euclid’s division lemma there exist integers q and r such that
a = 4q + r,
where 0 ≤ r < 4
⇒ a – 4q or 4q + 1 or 4q + 2 or 4q + 3
However since ‘a’ is odd we reject the case 4q and 4q + 2 as they both are divisible by 2. Therefore, any positive odd integer is of the form 4q +1 or 4q + 3.

Question 10.
Check whether 14ⁿ can end with the digit zero for any natural number n ? [CBSE Delhi 2016]
Answer:
Let, us suppose that 14ⁿ ends with the digit 0 for some n ∈ N
∴ 14ⁿ is divisible by 5
But, prime factors of 14 are 2 and 7.
∴ Prime factor of (14)ⁿ are (2 × 7)ⁿ
⇒ It is clear that in prime factorisation of 14″ there is no place for 5.
∴ By Fundamental theorem of Arithmetic
Every composite no. can be expressed as a product of primes and this factorisation is unique, a part from the order in which the prime factor occur.
∴ Our Supposition is wrong.
Hence, there exists no natural number n for which 14ⁿ ends with the digit zero.

Question 11.
Prove that √2 is an irrational number. [CBSE 2019]
Answer:
Let, if possible to the contrary that √2 is not irrational number i.e., √2. is a rational number.
That mean √2 can be expressed in \(\frac{p}{q}\) form where
p and q are coprime positive integers and q ≠ 0.
So, √2 = \(\frac{p}{q}\)
⇒ p² = 2q²
Thus, p² is a multiple of 2
⇒ p is a multiple of 2.
Let p = 2m for some integer m.
⇒ q² = 2m².
Thus, q² is a multiple of 2.
⇒ q is a multiple of 2.
Hence, 2 is a common factor of p and q.
This contradicts the fact that p and q are coprimes.
∴ Our supposition is wrong.
Hence, √2 is an irrational number.

Real Numbers Class 10 Extra Questions Long Answer Type 1

Question 1.
A, B and C starts cycling around a circular path in the same direction at the same time. Circumference of the path is 1980 m. If speed of A is 330 m/min, speed of B is 198 n0nin and that of C is 220 n^min and they start from the same point, then after what time will they be together at the starting point?
Answer:
As = \(\frac{\text { Distance }}{\text { Speed }}\)
Time taken by A to complete one round
= \(\frac{1980}{330}\) = 6 min
Time taken by B to complete one round
= \(\frac{1980}{198}\) = 10 min
Time taken by C to complete one round
= \(\frac{1980}{220}\) = 9 min
The three cyclists will be together after LCM (6, 10, 9)
6 = 2 × 3
10 = 2 × 5
9 = 3²
LCM (6, 10, 9) = 2¹ × 3² × 5 = 90 min.

Question 2.
The HCF of 408 and 1032 is expressible in the form 1032 m – 2040. Find the value of m. Also, find the LCM of 408 and 1032.
Answer:
Let us find HCF of 408 and 1032.
Here, 1032 > 408
∴ 1032 = 2 × 408 + 216
408 = 1 × 216 + 192
216 = 1 × 192 + 24
192 = 8 × 24 + 0
Thus, HCF of 408 and 1032 is 24.
Now, HCF (408, 1032)
i.e., 24 = 1032 × m – 2040
⇒ 1032 × m = 24 + 2040
⇒ 1032 × m = 2064
⇒ m = \(\frac{2064}{1032}\) = 2
408 = 2³ × 3 × 17
1032 = 2³ × 3 × 43 ,
∴ LCM of 408 and 1032 = 2³ × 3 × 17 × 43 = 17544.

Question 3.
Obtain the HCF of420 and 272 by using Euclid’s division algorithm and verify the same by using Fundamental theorem of Arithmetic. ‘
Answer:
Case I: Using Euclid’s division algorithm
420 = 272 × 1 + 148
272 = 148 × 1 + 124,
148 = 124 × 1 + 24
124 = 24 × 5 + 4 24 = 4×6 + 0
∴ HCF (420,272) = 4

Case II: Using Fundamental theorem of Arithmetic.

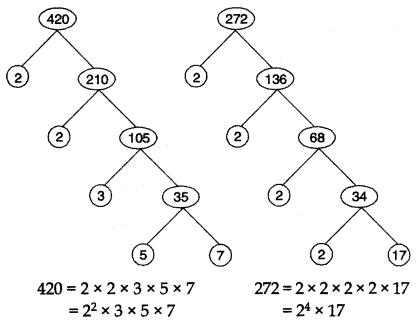
∴ HCF (420, 272) = 2² = 4
rom I and II the result obtained is verified.

Question 4.
If ‘h’ is HCF of 609 and 957. Find x and y satisfying h = 609x + 957y. Also show that x and y are not unique.
Answer:
Here 957 > 609
So, starting with
a = 957 and b = 609 and applying Euclid’s division algorithm,
we get
957 = 6 × 1 + 348 …………… (i)
609 = 348 × 1 + 261 …………… (ii)
348 = 261 × 1 + 87 …………… (iii)
261 = 87 × 3 + 0 ← remainder (r) …………… (iv)
⇒ HCF (609, 957) = 87
Alternatively,

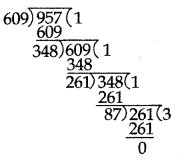
From (iii)
⇒ 87 = 348 – 261 × 1,
= 348 – (609 – 348 × 1) × 1
[∵ From (ii) 261 = 609 – 348 × 1]
= 348 – 609 × 1 + 348 × 1
= 348 × 2 – 609 × 1
= (957 – 609 × 1) × 2 – 609 × 1
= 957 × 2 – 609 × 2 – 609 × 1
= 957 × (2) + 609 (- 2 -1)
= 957 × (2) + 609 × (-3) …….. (iv)
Thus, 87 = 957y + 609x where y = 2 and x = – 3
Adding and subtracting 957 × 609 in (iv),
we get
87 = 957 × 2 + 609 × (- 3) + 957 × 609 – 957 × 609
= 957 × 2 + 957 × 609 + 609 × (-3) + 609 × (-957)
= 957 × (2 + 609) + 609 × (- 3 – 957)
⇒ 87 = 957 × (611) + 609 × (- 960) ………….. (v)
= 957x + 609y
Where x = 611, y = – 960
(v) represents another linear combination and hence x and y are not unique.

Real Numbers Class 10 Extra Questions HOTS

Question 1.
Find the greatest number that will divide 43,91 and 183 so as to leave the same remainder in each case.
Answer:
Here we look at the difference between the given numbers. Let the remainder in each case be x. then, 43 – x, 91 – x and 183 – x are divisible by the required number.
⇒ (91 – x) – (43 – x), (183 – x) – (91 – x) and (183 – x) – (43 – x) are divisible by the required number.
i.e., (91 – 43), (183 – 91) and (183 – 43) are divisible by the required number.
Required number
= HCF of (91 – 43), (183 – 91) and (183 – 43)
= HCF (48, 92,140)
As 48 = 2⁴ × 3,92 = 23 × 2²,
140 = 2² × 5 × 7
∴ HCF = 2² = 4
Thus, 4 is the greatest number that divides 43,91 and 183 leaving same remainder.

Question 2.
Coloured pencils are available in market in packs of 12 each and crayons in pack of 32 each. A girl wants full pack of both and the same number of pencils and crayons, how many of each would she need to buy ?
Answer:
LCM (12,32) = 96
∴ No. of packets of coloured pencils
= \(\frac{\text { LCM }}{\text { Qty. in one packed }}\) = \(\frac{96}{12}\) = 8
No. of packets of crayon = \(\frac{96}{32}\) = 3.

Question 3.
The set of Mathematics, Physics and Physical Education books have to be stacked in such a way that all the books are stored topic wise. The number of Mathematics, Physics and Physical Education books are 14, 18 and 22. Determine the number of stacks of each books provided books are of the same thickness.
Answer:
Firstly, to arrange the books as according to condition, find HCF of 14, 18 and 22.

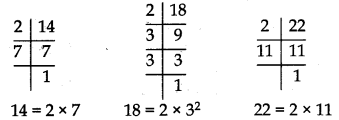
HCF (14, 18, 22) = 2
⇒ There are only 2 books in each stack.
Now, number of stacks of Mathematics books = \(\frac{14}{2}\) = 7
Number of stacks of Physics books = \(\frac{18}{2}\) = 9.
Number of stacks of Physical Education books = \(\frac{22}{2}\) = 11

Question 4.
Prove that there is no rational number whose square is 6.
Answer:
Let \(\frac{a}{b}\) be a rational number whose square is 6.
Then 6 = \(\left(\frac{a}{b}\right)^2\), where a and b are prime to each other and b > 0.
⇒ 6b² = a²
⇒ 3(2b²) = a²
Thus, a² is divisible by 3, and so a is divisible by 3.
Let a = 3p, where p is some integer.
Then, a² = 6b² gives
(3p)² = 6b²
⇒ 9 p² = 6b²
⇒ 3 p² = 2b²
This shows that 2 b² is divisible by 3, so b² is divisible by 3, and thus b is divisible by 3. Thus, both a and b are divisible by 3 which is a contradiction.
Hence, there is no rational number whose square is 6.

Question 5.
If p and q are two odd prime numbers, show that p2 – g2 is composite.
Answer:
Let p = 2m + 1, q = 2n + 1, where m and n are natural numbers.
Now, (p² – q²) = (p – q)(p + q) ,
But, p- q = (2m + 1) – (2n + 1) = 2(m – n)
p + q = (2m + 1) + (2n + 1) = 2 (m + n + 1)
Clearly both factors of (p² – q²) are even i.e., none of the divisors (p – q) and (p + q) is equal to 1.
⇒ (p² – q²) is a composite number.

Multiple Choice Questions

Choose the correct option out of four given in each of the following:

Question 1.
The value of H.C.F × L.C.M. for the number 183 and 30 is:
(a) 5490
(b) 610
(c) 61
(d) 549
Answer:
(a) 5490

Question 2.
Which of the following is not true about \(\frac{51}{1500}\)?
(a) It has a terminating decimal expansion
(b) It has a non-terminating decimal expansion
(c) It is a rational number which terminates after 5 places of decimal
(d) Its denominator, in lowest form, has prime factorisation of the form 2^m × 5ⁿ.
Answer:
(c) It is a rational number which terminates after 5 places of decimal

Question 3.
The decimal expansion of \(\frac{246}{2^7 \times 5^{-3}}\) terminates after
(a) 7 places of decimals
(b) 10 places of decimals
(c) 6 places of decimals
(d) Can’t be determined.
Answer:
(c) 6 places of decimals

Question 4.
The decimal expansion of
π = \(\frac{\text { Circumference of the circle }}{\text { diameter of circle }}\) is always
(a) terminating
(b) non-terminating, non repeating
(c) terminating repeating
(d) non terminating repeating.
Answer:
(b) non-terminating, non repeating

Question 5.
The least number divisible by 2,3,7 and 9 is:
(a) 126
(b) 256
(c) 251
(d) 189
Answer:
(a) 126

Question 6.
Let p/q be a rational number. Then, the condition on q such that p/q has a non terminating but repeating decimal expansion is
(a) q = 2^m × 5ⁿ; m, n are whole numbers
(b) q ≠ 2^m × 5ⁿ; m, n are whole numbers
(c) q = 2^m × 3ⁿ; m, n are whole numbers
(d) q ≠ 2^m × 3ⁿ; m, n are whole numbers
Answer:
(b) q ≠ 2^m × 5ⁿ; m, n are whole numbers

Question 7.
The decimal representation of an irrational number is always .
(a) terminating
(b) terminating, repeating
(c) non-terminating, repeating
(d) non-terminating, non repeating.
Answer:
(d) non-terminating, non repeating.

Question 8.
The H.C.F and L.C.M. of two numbers are 4 and 48. If one number is 12, then the other number is:
(a) 8
(b) 24
(c) 4
(d) 16
Answer:
(d) 16

Question 9.
According to Fundamental Theorem of Arithmetic, if p (a prime number) divides a2, and a is non-negative integer then:
(a) a divides p
(b) p divides a
(c) a² divides p
(d) p² divides a
Answer:
(b) p divides a

Question 10.
If the product of two coprime numbers is 217, then their L.C.M. is
(a) Can’t be determined
(b) 217
(c) 651
(d) 434
Answer:
(b) 217

Question 11.
Euclid’s division lemma states that for any two positive integers a and b there exist unique integers q and r such that a = bq + r, where r must satisfy
(a) 1 < r < b
(b) 0 < r ≤ b
(c) 0 ≤ r < b
(d) 0 < r < b
Answer:
(c) 0 ≤ r < b

Question 12.
For some integer p, every odd integer is of the form
(a) p
(b) p + 1
(c) 2p
(d) 2p + 1
Answer:
(d) 2p + 1

Question 13.
Which of the following is false:
(a) H.C.F. (a,b) × L.C.M.(a ,b) = a × b
(b) L.C.M. (p, q, r) = p × q × r; if p, q, r are prime numbers
(c) H.C.F. (p, q, r) = 1, if p, q, r are prime numbers
(d) H.C.F. (p, q, r) × L.C.M.(p, q, r) = p × q × r
Answer:
(d) H.C.F. (p, q, r) × L.C.M.(p, q, r) = p × q × r

Question 14.
Two integers p and q are expressed in powers of primes as p = a¹⁵b³ef⁷ and q = a⁴bgc² where a, b, c, d, e, f, g are primes. Then H.C.F. (p, q) is:
(a) a⁴bg
(b) a⁴b
(c) a¹⁵b³ef⁷c²
(d) a⁴bc²
Answer:
(b) a⁴b

Question 15.
The product of two consecutive natural numbers is always:
(a) an odd number
(b) an even number
(c) a prime number
(d) a composite number
Answer:
(b) an even number

Fill in the blanks:

Question 1.
If a number can’t be expressed in the form p/q, where p and q are integers and q ≠ 0, then it is known as ____________ number.
Answer:
Irrational

Question 2.
If a rational number p/q, where q ≠ 0 and p, q are coprimes, has a terminating decimal expansion then q has prime factors ___________ and ____________ .
Answer:
2 and 5

Question 3.
If a rational number piq (in lowest form) has a non-terminating and repeating decimal expansion, then prime factorisation of q is of the form ______________ .
Answer:
2^m × 5ⁿ, where m, n are non-negative integers

Question 4.
Euclid’s division algorithm is a technique to compute the ______________ of two given positive integers.
Answer:
H.C.F

Question 5.
H.C.F. of two or more prime numbers is always
Answer:
1

Question 6.
7 × 11 × 13+ 3 is a ____________ number, (prime/composite/irrational)
Answer:
Composite

Question 7.
If product of two coprimes is 765, then their L.C.M. = ____________.
Answer:
765

Question 8.
Sum of a rational and irrational number is always ____________ number.
Answer:
irrational

Question 9.
Product of any irrational number with a rational number ‘x’ is always rational. Then, x is ____________.
Answer:
0

Question 10.
Any number ending with ‘0’ must have ______________ and ______________ as its prime factors.
Answer:
2, 5

You can also download NCERT Solutions For Class 10 Maths to help you to revise the complete syllabus and score more marks in your examinations.

Extra Questions for Class 10 Maths

NCERT Solutions for Class 10 Maths