CBSE Sample Papers for Class 11 Maths Set 5 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 11 Maths with Solutions Set 5 are designed as per the revised syllabus.

CBSE Sample Papers for Class 11 Maths Set 5 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

• This Question paper contains five sections A, B, C, D, and E. Each section is compulsory. However, there are internal choices in some questions.
• Section A has 18 MCQs and 2 Assertion-Reason-based questions of 1 mark each.
• Section B has 5 Very Short Answer (VSA)-type questions of 2 marks each.
• Section C has 6 Short Answer (SA)-type questions of 3 marks each.
• Section D has 4 Long Answer (LA)-type questions of 5 marks each.
• Section E has 3 source-based/case-based/passage-based/integrated units of assessment of 4 marks each with sub-parts.

Section – A

(Multiple Choice Questions) Each Question Carries 1 Mark.

Question 1.
Let f(x) = \(\sqrt{1+x^2}\), then
(A) f(xy) = f(x).f(y)
(B) f(xy) ≥ f(x).f(y)
(C) f(xy) ≤ f(x).f(y)
(D) None of these
Answer:
(C) f(xy) ≤ f(x).f(y)
Explanation:

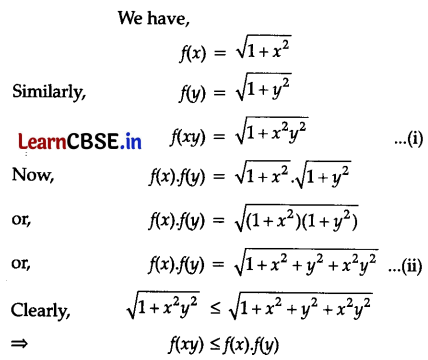
Question 2.
What is the length of the foot of the perpendicular drawn from the point P(3, 4, 5) on the Y-axis?
(A) \(\sqrt{41}\)
(B) \(\sqrt{34}\)
(C) 5
(D) None of these
Answer:
(B) \(\sqrt{34}\)
Explanation:
On y-axis, x = 0 and z = 0
Given, point P(3, 4, 5)
Let point A be (0, 4, 0)
Required distance, PA = \(\sqrt{(0-3)^2+(4-4)^2+(5-0)^2}\) = \(\sqrt{34}\) units

Question 3.
The set (A ∩ B’)’ ∪ (B ∩ C) is equal to
(A) A’ ∪ B ∪ C
(B) A’ ∪ B
(C) A’ ∪ C
(D) A’ ∩ B
Answer:
(B) A’ ∪ B
Explanation:
Since,
(A ∩ B)’ = A’ ∪ B’
∴ (A ∩ B’)’ ∪ (B ∩ C) = [A’ ∪ (B’)’] ∪ (B ∩ C)
= (A’ ∪ B) ∪ (B ∩ C)
= A’ ∪ B

Question 4.
The coefficient of xn in the expansion (1 + x + x² + ……..)^-n is
(A) 1
(B) (-1)ⁿ
(C) n
(D) n + 1
Answer:
(B) (-1)ⁿ
Explanation:
We know that
(1 + x + x2 + …..)-n = (1 – x)^-n
Now, the coefficient of xⁿ = (-1)ⁿ × ⁿC_n = (-1)ⁿ

Question 5.
If -3x + 17 < -13, then
(A) x ∈ (10, ∞)
(B) x ∈ [10, ∞)
(C) x ∈ [-∞, 10)
(D) x ∈ [-10, 10)
Answer:
(A) x ∈ (10, ∞)
Explanation:
Given, -3x + 17 < -13
Subtracting 17 from both sides,
⇒ -3x + 17 – 17 < -13 – 17
⇒ -3x < -30
⇒ x > 10 {since the division by a negative number inverts the inequality sign}
⇒ x ∈ (10, ∞)

Question 6.
If f(x) = \(\left(a-x^n\right)^{\frac{1}{n}}\), a > 0 and n ∈ N, then the value of f(f(x)) is
(A) \(\frac{1}{n}\)
(B) x
(C) x²
(D) \(x^{\frac{1}{2}}\)
Answer:
(B) x
Explanation:

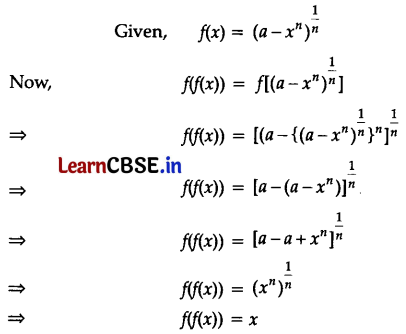
Question 7.
The sum of 10 items is 12 and the sum of their squares is 18. The standard deviation is
(A) \(\frac{1}{5}\)
(B) \(\frac{2}{5}\)
(C) \(\frac{3}{5}\)
(D) \(\frac{4}{5}\)
Answer:
(C) \(\frac{3}{5}\)
Explanation:

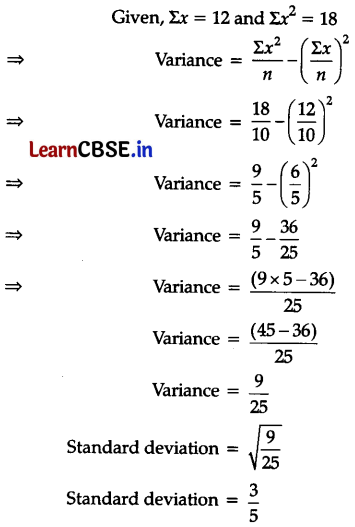
Question 8.
The locus of a point for which Y = 0 and Z = 0, is
(A) equation of X-axis
(B) equation of Y-axis
(C) equation of Z-axis
(D) None of these
Answer:
(A) equation of X-axis
Explanation:
The locus of a point for which y = 0 and z = 0 is the x-axis because on the x-axis, y = 0 and z = 0.

Question 9.
If f(x) = x sin x, then \(f^{\prime}\left(\frac{\pi}{2}\right)\) is equal to
(A) 0
(B) 1
(C) -1
(D) \(\frac{1}{2}\)
Answer:
(B) 1
Explanation:
Given, f(x) = x sin x
f'(x) = \(x\left[\frac{d}{d x} \sin x\right]+\sin x\left[\frac{d}{d x}(x)\right]\) = x cos x + sin x
Now, \(f^{\prime}\left(\frac{\pi}{2}\right)=\left(\frac{\pi}{2}\right) \cos \frac{\pi}{2}+\sin \frac{\pi}{2}\)
= \(\left(\frac{\pi}{2}\right)(0)+1\)
= 1

Question 10.
20 cards are numbered from 1 to 20. If one card is drawn randomly, what is the probability that the number on the card is a prime number?
(A) \(\frac{1}{5}\)
(B) \(\frac{2}{5}\)
(C) \(\frac{3}{5}\)
(D) 5
Answer:
(B) \(\frac{2}{5}\)
Explanation:
Let E be the event of getting a prime number.
E = {2, 3, 5, 7, 11, 13, 17, 19}
Hence, P(E) = \(\frac{8}{20}=\frac{2}{5}\)

Question 11.
If |x – 1| > 5, then
(A) x ∈ (-4, 6)
(B) x ∈ [-4, 6)
(C) x ∈ (-∞, -4) ∪ (6, ∞)
(D) x ∈ [-∞, -b) ∪ (b, ∞)
Answer:
(C) x ∈ (-∞, -4) ∪ (6, ∞)
Explanation:
We have, |x – 1| > 5
∴ (x – 1) < -5 or (x – 1) > 5
⇒ x < -5 + 1 or x > 5 + 1
⇒ x < -4 or x > 6
Thus, x ∈ (-∞, -4) ∪ (6, ∞)

Question 12.
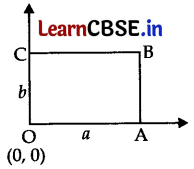
Let R be a set of points inside a rectangle of sides a and b (a, b > 1) with two sides along the positive direction of the x-axis and y-axis. Then
(A) R = {(x, y) : 0 ≤ x ≤ a, 0 ≤ y ≤ b}
(B) R = {(x, y) : 0 ≤ x < a, 0 ≤ y ≤ b}
(C) R = {(x, y) : 0 ≤ x ≤ a, 0 < y < b}
(D) R = {(x, y) : 0 < x < a, 0 < y < b}
Answer:
(D) R = {(x, y) : 0 < x < a, 0 < y < b}
Explanation:
Let R be the set of points a rectangle of sides a and b (a, b > 1) with two sides along the positive direction of the x-axis and y-axis.
R = {(x, y) : 0 < x < a and 0 < y < b}

Question 13.
The number of possible outcomes when a coin is tossed 6 times is
(A) 36
(B) 64
(C) 12
(D) 32
Answer:
(B) 64
Explanation:
Sample space of coins, S = {H, T}
n(S) = 2
No. of possible outcomes when a coin is tossed 6 times = 2⁶ = 64

Question 14.
If the middle term of \(\left(\frac{1}{x}+x \sin x\right)^{10}\) is equal to 7\(\frac{7}{8}\), then value of x is
(A) 2nπ + \(\frac{\pi}{6}\)
(B) nπ + \(\frac{\pi}{6}\)
(C) nπ + \((-1)^n \frac{\pi}{6}\)
(D) nπ + \((-1)^n \frac{\pi}{3}\)
Answer:
(C) nπ + \((-1)^n \frac{\pi}{6}\)
Explanation:
Here, n = 10, middle term 6th term
So, T₆ = T₅₊₁

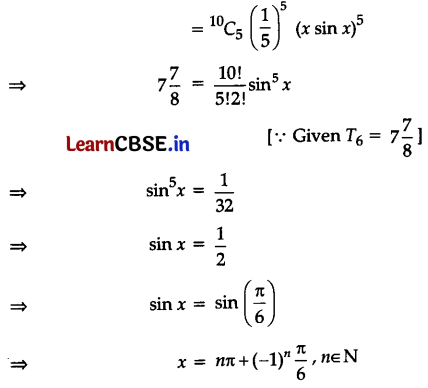
Question 15.
In how many ways can the word CHRISTMAS be arranged so that the letters C and M are never adjacent?
(A) 8! × (\(\frac{7}{2}\))
(B) 9! × (\(\frac{7}{2}\))
(C) 8! × (\(\frac{9}{2}\))
(D) None of these
Answer:
(A) 8! × (\(\frac{7}{2}\))
Explanation:
Words in which letters C and M are never adjacent = All cases – words having C and M together.
Total number of words = 9! ÷ 2! (as S is repeated)
Let us assume that C and M are one unit. Then, letters can be arranged in 8! ways.
C and M can be arranged in 2! ways.
Letter S is repeated, so the total number of ways will be divided by 2!
Number of words with C and M adjacent = 8! ÷ 2! × 2! = 8!
Words in which letters C and M are never adjacent = 9! ÷ 2! – 8!
= 8! × (\(\frac{9}{2}\) – 1)
= 8! × (\(\frac{7}{2}\))

Question 16.
The two successive terms in the expansion of (1 + x)²⁴ whose coefficients are in the ratio 1 : 4 are
(A) 3rd and 4th
(B) 4th and 5th
(C) 5th and 6th
(D) 6th and 7th
Answer:
(C) 5th and 6th
Explanation:
Let two successive terms in the expansion of the (1 + x)²⁴ be (r + 1)^th and (r + 2)^th terms.
⇒ \(\frac{{ }^{24} C_r}{{ }^{24} C_{r+1}}=\frac{1}{4}\)
⇒ \(\frac{r+1}{24-r}=\frac{1}{4}\)
⇒ 4r + 4 = 24 – r
⇒ r = 4
Therefore, T_r+1 = T₅ and T_r+2 = T₆
Hence, the 5th and 6th terms.

Question 17.
If A lies in the second quadrant and 3tan A + 4 = 0, then the value of (2cot A – 5cos A + sin A) is equal to
(A) \(\frac{-53}{10}\)
(B) \(\frac{23}{10}\)
(C) \(\frac{37}{10}\)
(D) \(\frac{7}{10}\)
Answer:
(B) \(\frac{23}{10}\)
Explanation:
Given that A lies in the second quadrant and 3tan A + 4 = 0.
3tan A = -4
tan A = \(\frac{-4}{3}\)
cot A = \(\frac{1}{\tan A}=\frac{-3}{4}\)
Using the identity sec²A = 1 + tan²A,

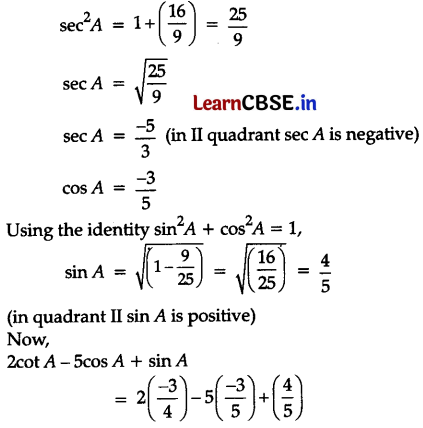
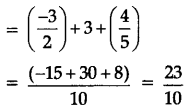
Question 18.
The modulus of 5 + 4i is
(A) 41
(B) -41
(C) \(\sqrt{41}\)
(D) \(\sqrt{-41}\)
Answer:
(C) \(\sqrt{41}\)
Explanation:
Let z = 5 + 4i
Now, the modulus of z is calculated as:
|z| = \(\sqrt{\left(5^2+4^2\right)}\)
⇒ |z| = \(\sqrt{(25+16)}\)
⇒ |z| = \(\sqrt{41}\)

Assertion-Reason Based Questions
In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct answer out of the following choices.

(A) Both (A) and (R) are true and (R) is the correct explanation of (A).
(B) Both (A) and (R) are true but (R) is not the correct explanation of (A).
(C) (A) is true but (R) is false.
(D) (A) is false but (R) is true.

Question 19.
Assertion (A): The degree measure corresponding to (-2) radian is -114°19′.
Reason (R): The degree measure of a given radian measure = \(\frac{180^{\circ}}{\pi}\) × radian measure
Answer:
(D) (A) is false but (R) is true.
Explanation:
Degree Measure of (-2) radian

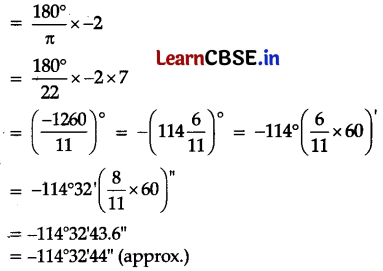
Question 20.
Assertion (A): The greatest integral value of λ for which (2λ – 1)x² – 4x + (2λ – 1) = 0 has real roots, is 2.
Reason (R): For real roots of ax² + bx + c = 0, D ≥ 0
Answer:
(D) (A) is false but (R) is true.
Explanation:
For real roots D ≥ 0
⇒ (-4)² – 4(2λ – 1)(2λ – 1) ≥ 0
⇒ (2λ -1)² ≤ 4
⇒ -2 ≤ 2λ – 1 ≤ 2
⇒ \(-\frac{1}{2} \leq \lambda \leq \frac{3}{2}\)
Hence, the greatest integral value of λ = 1

Section-B

[This section comprises very short answer-type questions (VSA) of 2 marks each]

Question 21.
Prove that cos 510° cos 330° + sin 390° cos 120° = -1.
OR
Find the domain of the relation, R = {(x, y) : x, y ∈ Z, xy = 4}.
Answer:
The values must be the following:
cos 510°= cos(90° × 5 + 60°) = -sin 60° = \(\frac{-\sqrt{3}}{2}\)
cos 330° = cos(90° × 3 + 60°) = sin 60° = \(\frac{\sqrt{3}}{2}\)
sin 390° = sin(90° × 4 + 30°) = sin 30° = \(\frac{1}{2}\)
cos 120° = cos(90° × 1 + 30°) = -sin 30° = \(\frac{-1}{2}\)
Now, L.H.S. = cos 510° cos 330° + sin 390° cos 120°
= \(\left(\frac{-\sqrt{3}}{2}\right)\left(\frac{\sqrt{3}}{2}\right)+\left(\frac{1}{2}\right)\left(\frac{-1}{2}\right)\)
= \(\frac{-3}{4}+\left(\frac{-1}{4}\right)\)
= -1
= R.H.S.
Hence proved.
OR
Given, R = {(x, y) : x, y ∈ Z, xy = 4}
= {(-4, -1), (-2, -2), (-1, -4), (1, 4), (2, 2), (4, 1)}
∴ Domain of R = {-4, -2, -1, 1, 2, 4}

Question 22.
Find the derivative of sin 2x by the first principle.
Answer:
Let f(x) = sin 2x
∴ By first principle

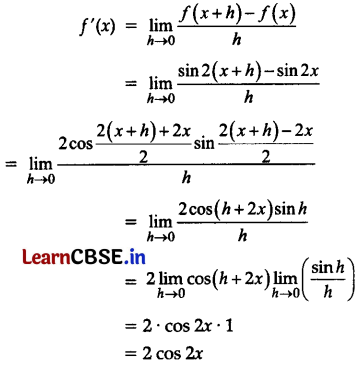
Question 23.
If the coefficient of second, third, and fourth terms in the expansion of (1 + x)²ⁿ are in A.P. then show that 2n² – 9n = -7.
OR
In how many ways can the letters of the word “PENCIL” be arranged so that I is always next to L?
Answer:
The general term of (1 + x)²ⁿ is

⇒ 6(2n – 1) = 6 + 4n² – 2n – 4n + 2
⇒ 12n – 6 = 8 + 4n² – 6n
⇒ 4n² – 6n – 12n = -14
⇒ 2n² – 9n = -7 [dividing by 2]
Hence proved.
OR
There are 6 letters in the word “PENCIL”.
Consider LI as one letter. Now 5 letters (P, E, N, C, I, L) can be arranged in ⁵P₅ = 5! = 120 ways.
Hence, the total number of ways in which I is always next to L is 120.

Question 24.
Solve the equation, 4x – 2 ≤ 6 and 9x + 3 ≥ -15.
Answer:
First 4x – 2 ≤ 6
⇒ 2x – 1 ≤ 3
⇒ 2x ≤ 4
⇒ x ≤ 2
Now, 9x + 3 ≥ -15
⇒ 3x + 1 ≥ -5
⇒ 3x ≥ -6
⇒ x ≥ -2
Hence, the solution is [-2, 2]

Question 25.
If A and B are two events having P(A ∪ B) = \(\frac{1}{2}\) and P(\(\bar{A}\)) = \(\frac{2}{3}\), find P(B ∩ \(\bar{A}\)).
Answer:

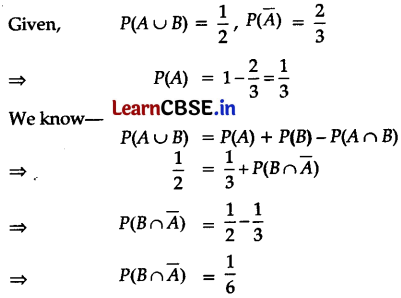
Section-C

[This section comprises short answer type questions (SA) of 3 marks each]

Question 26.
Find sets A, B, and C such that A ∩ B, B ∩ C, and A ∩ C are non-empty sets and A ∩ B ∩ C = φ.
Answer:
Let take three sets A = {1, 2}, B = {2, 3} and C = {3, 1}
A ∩ B, B ∩ C and A ∩ C should be nonempty sets.
A ∩ B = {2}, B ∩ C = {3} and A ∩ C = {1}
Therefore, A ∩ B, B ∩ C, and A ∩ C are non-empty.
The intersection of all three sets is a null set, A ∩B ∩ C = φ.

Question 27.
Find the equation of the line parallel to the line 3x – 4y + 2 = 0 and pass through the point (-2, 3).
Answer:
The given line is 3x – 4y + 2 = 0
⇒ 4y = 3x + 2
⇒ y = \(\frac{3}{4} x+\frac{1}{2}\)
∴ Slope, m = \(\frac{3}{4}\)
∴ Slope of the parallel line is m = \(\frac{3}{4}\)
Hence, the equation of a line with slope \(\frac{3}{4}\) and passing through (-2, 3) is y – 3 = \(\frac{3}{4}\)(x + 2)
⇒ 3x + 6 = 4y – 12
⇒ 3x – 4y + 18 = 0 is the required equation of the line.

Question 28.
How many liters of water will have to be added to 1250 liters of 45% solution of acid so that the resulting mixture will contain more than 25% but less than 30% acid content?
OR
If A = 1 + ra + r²a + …….. ∞, then express r in terms of ‘a’ and ‘A’.
Answer:
Let x liter of water is required to be added.
Then, total mixture = (x + 1250) litres
The amount of acid contained in the resulting mixture is 45% of 1250 liters.
This resulting mixture will contain more than 25% but less than 30% acid content.
∴ 30% of (1250 + x) > 45% of 1250
And, 25% of (1250 + x) < 45% of 1250
⇒ \(\frac{30}{100}\) (1250 + x) > \(\frac{45}{100}\) × 1250
⇒ 30(1250 + x) > 45 × 1250
⇒ 30 × 1250 + 30x > 45 × 1250
⇒ 30x > 45 × 1250 – 30 × 1250
⇒ 30x > (45 – 30) × 1250
⇒ x > 625
25% of (1250 + x) < 45% of 1250
⇒ \(\frac{25}{100}\) (1250 + x) < \(\frac{45}{100}\) × 1250
⇒ 25(1250 + x) < 45 × 1250
⇒ 25 × 1250 + 25x < 45 × 1250
⇒ 25x < 45 × 1250 – 25 × 1250
⇒ 25x < (45 – 25) × 1250
⇒ x < 1000
⇒ 625 < x < 1000
Thus, the required number of liters of water that is to be added will have to be more than 625 liters but less than 1000 liters.
OR
Given series is in G.P. with common ratio r^a.
∵ Sum of infinite terms of G.P. = \(\frac{x}{1-R}\)
where x is the first term and R is the common ratio.

Question 29.
Find the equation of circle concentric with circle 4x² + 4y² – 12x – 16y – 21 = 0 and half its area.
OR
If A(0, 4, 1), B(2, 3, -1) and C(4, 5, 0) are the vertices of triangle ABC, then show that ΔABC is a right-angled triangle.
Answer:
The given equation of the circle is



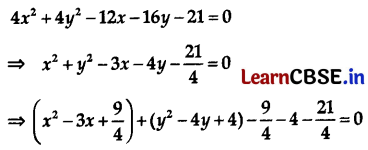
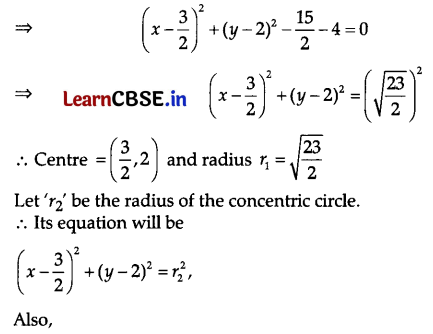
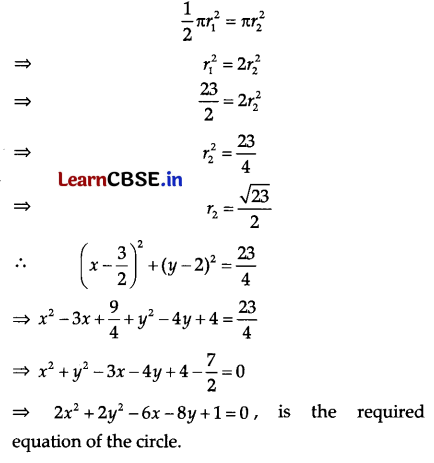
OR
The given points are A(0, 4, 1), B(2, 3, -1) and C(4, 5, 0)
AB² = (0 – 2)² + (4 – 3)² + (1 + 1)² = 4 + 1 + 4 = 9
BC² = (2 – 4)² + (3 – 5)² + (-1 – 0)² = 4 + 4 + 1 = 9
CA² = (4 – 0)² + (5 – 4)² + (0 – 1)² = 16 + 1 + 1 = 18
∴ AB² + BC² = CA²
Hence, ΔABC is a right-angled triangle.

Question 30.
A young man visits a hospital for a medical check-up. The probability that he has lung problem is 0.55, heart problem is 0.29 and either lungs or heart problem is 0.57. What is the probability that he has
(i) both types of problems lungs as well as heart?
(ii) lungs problem but not heart problem?
Out of 100 persons, how many are expected to have both types of problems?
OR
A card is drawn from a deck of 52 cards. Find the probability of getting a king a heart or a red card.
Answer:
Let ‘L’ and ‘H’ be the events that the young man has lung problems and heart problems respectively.
∴ P(L) = 0.55, P(H) = 0.29, P(L ∪ H) = 0.57, P(L ∩ H) = ?
(i) We know that,
P(L ∪ H) = P(L) + P(H) – P(L ∩ H)
⇒ P(L ∩ H) = P(L) + P(H) – P(L ∪ H)
= 0.55 + 0.29 – 0.57
= 0.27
∴ The probability that he has both problems is 0.27.
(ii) P(L ∩ H’) = P(L) – P(L ∩ H)
= 0.55 – 0.27
= 0.28
∴ The probability that he has a lung problem but not a heart problem is 0.28.
∴ Out of 1000 persons, the number of persons with both problems = 0.27 × 1000 = 270.
OR
Let ‘S’ be the sample space. Then, n(S) = 52.
Let A, B, and C be the events of getting a king, a heart, and a red card respectively.
Then, n(A) = 4, n(B) 13, n(C) = 26

Thus, the probability of getting a king or a heart or a red card \(\frac{7}{13}\).

Question 31.
A = {1, 2, 3, 4, 5}, S = {(x, y) : x ∈ A, y ∈ A}, then find the ordered pairs which satisfy the conditions given below.
(i) x + y = 5
(ii) x + y < 5
(iii) x + y > 8
Answer:
Given, A = {1, 2, 3, 4, 5} and S = {(x, y) : x ∈ A, y ∈ A}
(i) The set of ordered pairs satisfying x + y = 5 is {(1, 4), (2, 3), (3, 2), (4, 1)}.
(ii) The set of ordered pairs satisfying x + y < 5 is {(1, 1), (1, 2), (1, 3), (2, 1), (2, 2), (3, 1)}.
(iii) The set of ordered pairs satisfying x + y > 8 is {(4, 5), (5, 4), (5, 5)}

Section-D

[This section comprises long answer type questions (LA) of 5 marks each]

Question 32.
The complex number z₁, z₂, z₃ satisfying \(\frac{z_1-z_3}{z_2-z_3}=\frac{1-i \sqrt{3}}{2}\) are the vertices of an equilateral triangle.
Answer:

Question 33.
Determine a quadratic function (f) that is defined by f(x) = ax² + bx + c. If f(0) = 6, f(2) = 1, f(-3) = 6.
OR
Prove that 2 sin²β + 4 cos(α + β) sin α sin β + cos 2(α + β) = cos 2α
Answer:
Given, f(x) = ax² + bx + c
At x = 0, f(0) = 6 (given)
∴ a × 0 + b × 0 + c = 6
⇒ c = 6 ……(i)
At x = 2, f(2) = 1 (given)
a(2)² + b(2) + c = 1
⇒ 4a + 2b + 6 = 1 (using (i))
⇒ 4a + 2b = -5 …..(ii)
At x = -3, f(-3) = 6
⇒ a(-3)² + b(-3) + c = 6
⇒ 9a – 3b + 6 = 6 (using (i))
⇒ 9a – 3b = 0 ……(iii)
On solving equations (ii) and (iii), we get
a = \(\frac{-1}{2}\) and b = \(\frac{-3}{2}\)
∴ Required quadratic function (f) = \(\frac{-1}{2} x^2+\frac{-3}{2} x+6\)
OR
L.H.S. = 2 sin²β + 4 cos(α + β) sin α sin β + cos 2(α + β)
= 2 sin²β + 4(cos α cos β – sin α sin β) sin α sin β + (cos 2α cos 2β – sin 2α sin 2β)
= 2 sin²β + 4 sin α cos α sin β cos β – 4 sin²α sin²β + cos 2α cos 2β – sin 2α sin 2β
= 2 sin²β + sin 2α sin 2β – 4 sin²α sin²β + cos 2α cos 2β – sin 2α sin 2β
= (1 – cos 2β) – (2 sin²α)(2 sin²β) + cos 2α cos 2β
= (1 – cos 2β) – (1 – cos 2α) (1 – cos 2β) + cos 2α cos 2β
= 1 – cos 2β – (1 – cos 2β – cos 2α + cos 2α cos 2β) + cos 2α cos 2β
= cos 2α
= R.H.S.
Hence Proved.

Question 34.
Calculate the mean deviation about mean:

Marks	0-10	10-20	20-30	30-40	40-50
No. of Students	6	7	15	16	4

Answer:

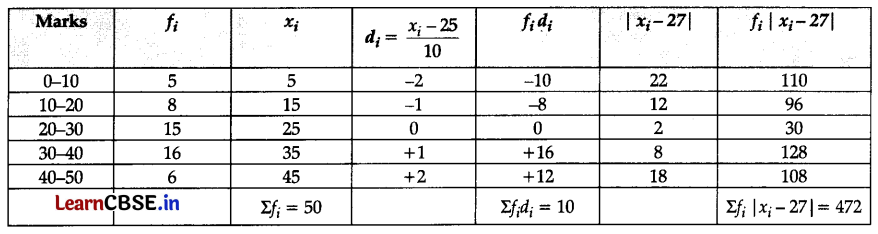
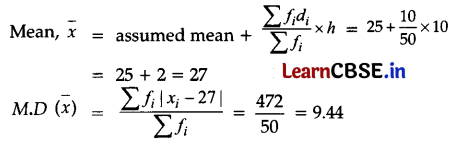
Question 35.
\(\lim _{x \rightarrow \pi} \frac{1-\sin \frac{x}{2}}{\cos \frac{x}{2}\left(\cos \frac{x}{4}-\sin \frac{x}{4}\right)}\)
OR
If f(x) = \(\left\{\begin{array}{cc}
x+2, & x \leq-1 \\
c x^2, & x \geq-1
\end{array}\right.\), then find c when \(\lim _{x \rightarrow-1} f(x)\) exists.
Answer:

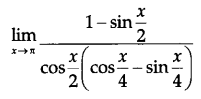
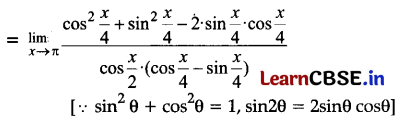
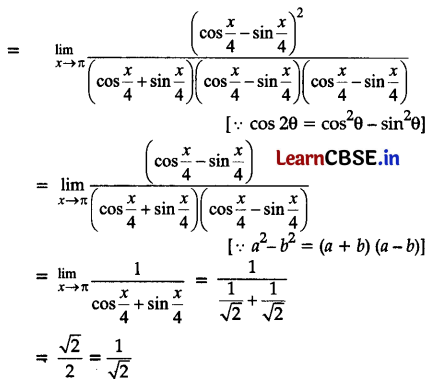
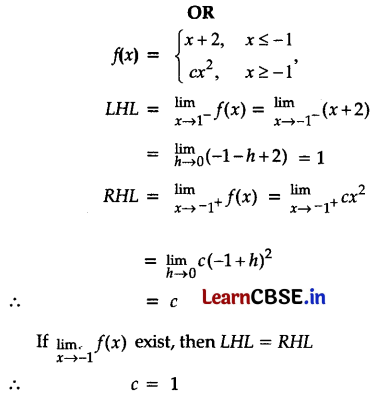
Section-E

[This section comprises 3 case-study/passage-based questions of 4 marks each with sub-parts.]

The first two case study questions have three sub-parts (i), (ii), and (iii) of marks 1, 1, and 2 respectively. The third case study question has two sub-parts of 2 marks each.

Question 36.
Read the following passage and answer the questions given below:
A company produces certain items. The manager in the company used to make a data record daily about the cost and revenue of these items separately. A product’s cost and revenue functions are given by C(x) = 20x + 4000 and R(x) = 60x + 2000, respectively, where x is the number of items produced and sold.

(i) How many items must be sold to realize some profit?
(ii) If the cost and revenue function of a product is given by C(x) = 2x + 400 and R(x) = 6x + 20 respectively, where x is the number of items produced by the manufacturer. Then find the minimum number of items that the manufacturer must sell to realise some profit.
(iii) Solve for x: 12x + 7 < -11.
OR
Solve for x: 5x – 8 > 40.
Answer:
(i) Here, R(x) > C(x)
⇒ 60x + 2000 > 20x + 4000
⇒ 40x > 2000
⇒ x > 50
Thus, to make some profit minimum of 51 items must be sold.
(ii) Here, R(x) > C(x)
⇒ 6x + 20 > 2x + 400
⇒ 4x > 380
⇒ x > 95
Thus, minimum items to make a profit = 96
(iii) 12x + 7 < -11
⇒ 12x < -11 – 7
⇒ 12x < -18
⇒ x < \(\frac{-3}{2}\)
OR
5x – 8 > 40
⇒ 5x > 48
⇒ x > \(\frac{48}{5}\)

Question 37.
Read the following passage and answer the questions given below:
Four friends Rishabh, Shubham, Vikram, and Rajkumar are sitting on vertices of a rectangle, whose coordinates are given.

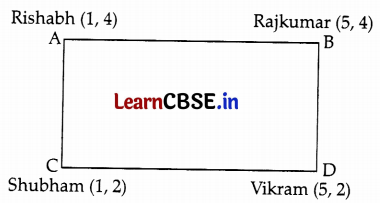
(i) Write the equation formed by Shubham and Rajkumar.
(ii) Write the equation formed by Rishabh and Vikram.
(iii) Write the intersection of the two lines formed above.
OR
Find the slope of the equation of the line formed by Rishabh and Rajkumar.
Answer:
(i) We have the positions,
Shubham B(1, 2) and Rajkumar D(5, 4)
Slope, m1 = \(\frac{4-2}{5-1}=\frac{2}{4}=\frac{1}{2}\)
Taking point (1, 2) = (x₁, y₁) and m₁ = \(\frac{1}{2}\)
The equation of the line joining points B and D is (y – y₁) = m₁ (x – x₁)
⇒ (y-2) = \(\frac{1}{2}\) (x – 1)
⇒ 2y – 4 = x – 1
⇒ x – 2y + 3 = 0
(ii) We have the positions, Rishabh A(1, 4) and Vikram C(5, 2)
Slope, m₂ = \(\frac{2-4}{5-1}=\frac{-2}{4}=-\frac{1}{2}\)
Taking point (x₁, y₁) = (1, 4) and m₂ = \(\frac{-1}{2}\)
Equation of line is (y – 4) = \(\frac{-1}{2}\)(x – 1)
⇒ 2y – 8 = -x + 1
⇒ x + 2y – 9 = 0
(iii) We have, x – 2y + 3 = 0 …….(i)
and x + 2y – 9 = 0 ……..(ii)
On adding Equations (i) and (ii), we get
2x – 6 = 0
⇒ x = 3
∴ 3 + 2y – 9 = 0
⇒ 2y = 6
⇒ y = 3
Hence, point of intersection is (3, 3).
OR
We have positions,
Rishabh A(1, 4) and Rajkumar D(5, 4)
Slope AD = \(\frac{4-4}{5-1}\) = 0
Hence, the slope is zero.

Question 38.
Read the following passage and answer the questions given below:
If Sangeeta has set A and Shamma has set B such that n(A) + n(B) = 9. The number of elements of the power set of set A is 8 times the number of elements of the power set of set B.
(i) Find n(A).
(ii) Find n(B).
Answer:
(i) We know that, n(P(A)) = 2^n(A) and n(P(B)) = 2^n(B)
So \(\frac{2^{n(A)}}{2^{n(B)}}=\frac{8}{1}\)
⇒ \(2^{n(A)-n(B)}\) = 8
⇒ \(2^{n(A)-n(B)}\) = 2³
⇒ n(A) – n(B) = 3 …..(i)
Given, n(A) + n(B) = 9 …(ii)
On solving (i) and (ii),
We get n(A) = 6
(ii) Given, n(A) + n(B) = 9 …..(ii)
And n(A) – n(B) = 3 …..(i) (from above)
Subtracting (i) from (ii)
2n(B) = 6
⇒ n(B) = 3